First we find the volume at point
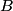 . We are told that the process BC is isothermal, so by the ideal gas law . We are told that the process BC is isothermal, so by the ideal gas law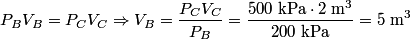 The work done by the gas as it goes from state 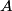 to state to state 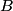 is is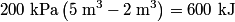 The work done by the gas as it goes from state 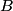 to state to state 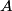 is is The negative sign indicates that work is actually being done on the gas. During the process C to A, there is not net work done on the gas because 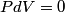 . Therefore, the net work done by the gas during the complete cycle beginning and ending at A is . Therefore, the net work done by the gas during the complete cycle beginning and ending at A is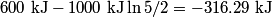 Therefore, answer (D) is correct. |
Please send questions or comments to X@gmail.com where X = physgre.